Length of the Tangent from a Point to a Circle
The length of tangent from the point to the circle is
Image of the Circle by the Line Mirror
Let the circle be and line mirror is in this condition, radius of circle remains unchanged but centres changes. Let the centre of imaged circle be .
Then,
we get,
and

Then,
we get,
and

Common Chord of Two Circles
The equation of common chord of two circles
$$ S \equiv x^2+y^2+2 g x+2 f y+c=0 $$
and $ S^{\prime} \equiv x^2+y^2+2 g^{\prime} x+2 f^{\prime} y+c^{\prime}=0$ is
$ 2 x\left(g-g^{\prime}\right)+2 y\left(f-f^{\prime}\right)+c-c^{\prime}=0$ i.e.
$ S-S^{\prime}=0$

Length of common chord :
We have, $\quad P Q=2(P M) \quad(\because M$ is mid-point of $P Q)$
$$ =2 \sqrt{\left\{\left(C_1 P\right)^2-\left(C_1 M\right)^2\right\}} $$
where, $C_1 P=$ radius of the circle $(S=0)$
and $C_1 M=$ length of perpendicular from $C_1$ on common chord $P Q$.
$$ S \equiv x^2+y^2+2 g x+2 f y+c=0 $$
and $ S^{\prime} \equiv x^2+y^2+2 g^{\prime} x+2 f^{\prime} y+c^{\prime}=0$ is
$ 2 x\left(g-g^{\prime}\right)+2 y\left(f-f^{\prime}\right)+c-c^{\prime}=0$ i.e.
$ S-S^{\prime}=0$

Length of common chord :
We have, $\quad P Q=2(P M) \quad(\because M$ is mid-point of $P Q)$
$$ =2 \sqrt{\left\{\left(C_1 P\right)^2-\left(C_1 M\right)^2\right\}} $$
where, $C_1 P=$ radius of the circle $(S=0)$
and $C_1 M=$ length of perpendicular from $C_1$ on common chord $P Q$.
When two Circles Touch each other Internally
Then, distance between their centres Difference of their radii


When two circles touch each other externally
Then, distance between their centres $=$ sum of their radii i.e.
$\left|C_1 C_2\right|=r_1+r_2$

$\left|C_1 C_2\right|=r_1+r_2$

Director Circle
The equation of the director circle of the circle is
Pair of Tangents
The combined equation of the pair of tangents drawn from a point $P\left(x_1, y_1\right)$ to the circle $x^2+y^2=a^2$ is
$$ \left(x^2+y^2-a^2\right)\left(x_1^2+y_1^2-a^2\right)=\left(x x_1+y y_1-a^2\right)^2 $$
or $ S S_1=T^2$
where, $ S=x^2+y^2-a^2, S_1=x_1^2+y_1^2-a^2$
and $ T=x x_1+y y_1-a^2$
$$ \left(x^2+y^2-a^2\right)\left(x_1^2+y_1^2-a^2\right)=\left(x x_1+y y_1-a^2\right)^2 $$
or $ S S_1=T^2$
where, $ S=x^2+y^2-a^2, S_1=x_1^2+y_1^2-a^2$
and $ T=x x_1+y y_1-a^2$
Chord Bisected at a Given Point
The equation of the chord of the circle bisected at the point is given by
or
or
Chord of Contact
The equation of the chord of contact of tangents drawn from a point to the
circle is
circle is
Power of a Point With Respect to a Circle
The length of tangent from the point to the circle is
Equation of Circle with Centre (h, k) and Radius r
The equation of circle is
Normal to a Circle at a Given Point
1. Point form :
The equation of normal at the point to the circle is
2. Parametric form :
Since, parametric coordinates of circle is .
Equation of normal at is
or or
or , where
which is slope form of normal.
The equation of normal at the point to the circle is
2. Parametric form :
Since, parametric coordinates of circle is .
Equation of normal at is
or or
or , where
which is slope form of normal.
Tangent to a Circle at a Given Point
1. Point form :
The equation of tangent at the point to a circle
2. Parametric form :
The equation of tangent to the circle at the point is
3. Slope form :
The equation of a tangent of slope to the circle is
and the coordinates of the point of contact are
The equation of tangent at the point to a circle
2. Parametric form :
The equation of tangent to the circle at the point is
3. Slope form :
The equation of a tangent of slope to the circle is
and the coordinates of the point of contact are
The Length of Intercept Cut-off from a Line by a Circle
The length of the intercept cut-off from the line $y=m x+c$ by the circle $x^2+y^2=a^2$ is
$$ 2 \sqrt{\left\{\frac{a^2\left(1+m^2\right)-c^2}{\left(1+m^2\right)}\right\}} $$
$$ 2 \sqrt{\left\{\frac{a^2\left(1+m^2\right)-c^2}{\left(1+m^2\right)}\right\}} $$
Position of a Point with Respect to Circle
A point lies outside, on or inside a circle
according as , =, or
where, .
according as , =, or
where, .
Intercepts Made on the Axes by a Circle
Let the circle
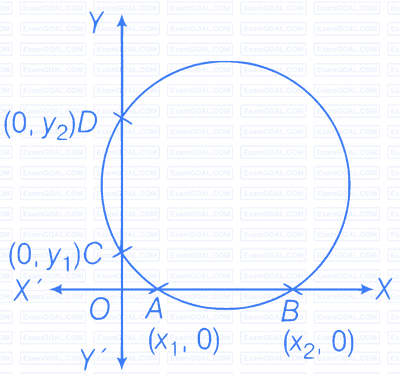

Diametric Form of a Circle
The equation of the circle on the line segment joining and as diameter is
Locus of the Mid-point of the Chords of the Circle that Subtends an Angle of 2θ at its Centre
Let mid-point and centre, radius of circle are respectively, then
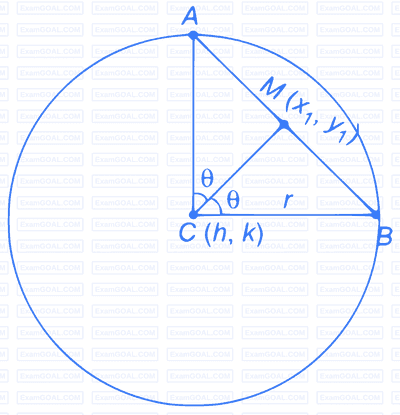
Required locus is

Required locus is
General Equation of a Circle
The general equation of circle is
$$ x^2+y^2+2 g x+2 f y+c=0, $$ where $g, f$ and $c$ are constants.
Here, Co-ordinates of the centre are $(-g,-f)$ and
radius $=\sqrt{g^2+f^2-c},\left(g^2+f^2 \geq c\right)$
$$ x^2+y^2+2 g x+2 f y+c=0, $$ where $g, f$ and $c$ are constants.
Here, Co-ordinates of the centre are $(-g,-f)$ and
radius $=\sqrt{g^2+f^2-c},\left(g^2+f^2 \geq c\right)$