Equation of Conic Section
If the focus is and the directrix is , then the equation of the conic section
whose eccentricity is
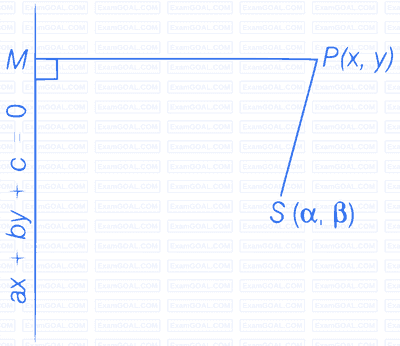
.
whose eccentricity is

.
Standard Equation of Parabola
Consider the focus of the parabola as and directrix be , and axis as -axis.

Now according to the definition of the parabola, for any point on the parabola, we must have
Vertex :
Tangent at vertex :
Equation of latus rectum :
Extremities of latus rectum :
Length of latus rectum :
Focal distance (SP) :
Parametric form : and , where is parameter

Now according to the definition of the parabola, for any point on the parabola, we must have
Vertex :
Tangent at vertex :
Equation of latus rectum :
Extremities of latus rectum :
Length of latus rectum :
Focal distance (SP) :
Parametric form : and , where is parameter
Other forms of Parabola with Latusrectum 4a
(1) Parabola opening to left (i.e.

(i) Vertex is .
(ii) Focus is .
(iii) Equation of the directrix is .
(iv) Equation of the axis is i.e. -axis.
(v) Equation of the tangent at the vertex is i.e. Y-axis.
(vi) Length of latusrectum .
(vii) Ends of latusrectum are and .
(viii) Equation of latusrectum is i.e. .
(ix) Parametric coordinates is .
(2) Parabola opening upwards (i.e.
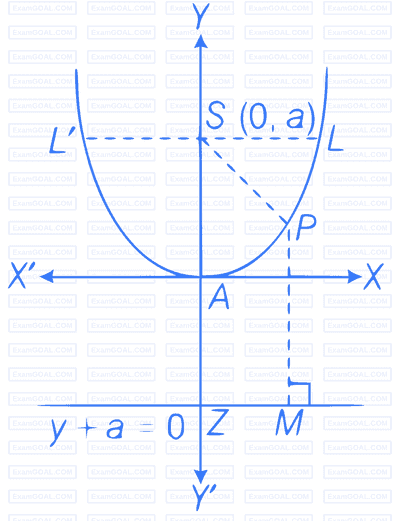
(i) Vertex is .
(ii) Focus is .
(iii) Equation of the directrix is .
(iv) Equations of the axis is i.e. -axis.
(v) Equation of the tangent at the vertex is i.e. -axis.
(vi) Ends of latusrectum are and .
(vii) Length of latusrectum .
(viii) Equation of latusrectum is i.e. .
(ix) Parametric coordinates is .
(3) Parabola opening downwards (i.e.
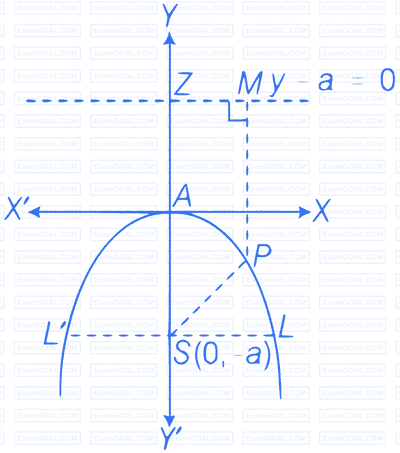
(i) Vertex is . (ii) Focus is .
(iii) Equation of the directrix is .
(iv) Equation of the axis is i.e. -axis.
(v) Equation of the tangent at the vertex is i.e. -axis.
(vi) Length of latusrectum
(vii) Ends of latusrectum are and
(viii) Equation of latusrectum is i.e. .
(ix) Parametric coordinates are .

(i) Vertex is .
(ii) Focus is .
(iii) Equation of the directrix is .
(iv) Equation of the axis is i.e. -axis.
(v) Equation of the tangent at the vertex is i.e. Y-axis.
(vi) Length of latusrectum .
(vii) Ends of latusrectum are and .
(viii) Equation of latusrectum is i.e. .
(ix) Parametric coordinates is .
(2) Parabola opening upwards (i.e.

(i) Vertex is .
(ii) Focus is .
(iii) Equation of the directrix is .
(iv) Equations of the axis is i.e. -axis.
(v) Equation of the tangent at the vertex is i.e. -axis.
(vi) Ends of latusrectum are and .
(vii) Length of latusrectum .
(viii) Equation of latusrectum is i.e. .
(ix) Parametric coordinates is .
(3) Parabola opening downwards (i.e.

(i) Vertex is . (ii) Focus is .
(iii) Equation of the directrix is .
(iv) Equation of the axis is i.e. -axis.
(v) Equation of the tangent at the vertex is i.e. -axis.
(vi) Length of latusrectum
(vii) Ends of latusrectum are and
(viii) Equation of latusrectum is i.e. .
(ix) Parametric coordinates are .
General Equation of a Parabola
Let be the focus, and is the equation of the directrix. Let be any point on the parabola.
Then by definition
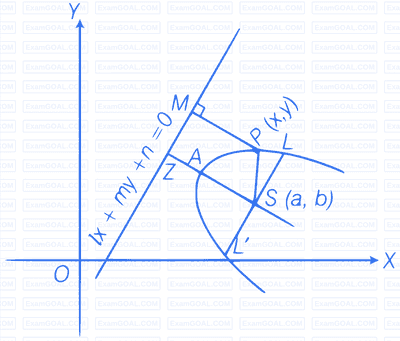
term term constant
This is of the form .
This equation is the general equation of parabola.
Then by definition

term term constant
This is of the form .
This equation is the general equation of parabola.
The Generalised form (y-k)2=4a(x-h)
The parabola
$$ y^2=4 a x $$
can be written as $(y-0)^2=4 a(x-0)$.
The vertex of this parabola is $A(0,0)$

Now, when origin is shifted at $A^{\prime}(h, k)$ without changing the direction of axes, its equation becomes
$$ (y-k)^2=4 a(x-h) $$
Note : 1. Another form is $(x-h)^2=4 a(y-k)$ axis parallel to $Y$-axis with its vertex $(h, k)$ its focus is at $(h, a+k)$ and length of latusrectum $=4 a$, the equation of the directrix is
$$ y=k-a \Rightarrow y+a-k=0 . $$
2. The parametric equation of $(y-k)^2=4 a(x-h)$ are $x=h+a t^2$ and $y=k+2 a t$.
$$ y^2=4 a x $$
can be written as $(y-0)^2=4 a(x-0)$.
The vertex of this parabola is $A(0,0)$

Now, when origin is shifted at $A^{\prime}(h, k)$ without changing the direction of axes, its equation becomes
$$ (y-k)^2=4 a(x-h) $$
Note : 1. Another form is $(x-h)^2=4 a(y-k)$ axis parallel to $Y$-axis with its vertex $(h, k)$ its focus is at $(h, a+k)$ and length of latusrectum $=4 a$, the equation of the directrix is
$$ y=k-a \Rightarrow y+a-k=0 . $$
2. The parametric equation of $(y-k)^2=4 a(x-h)$ are $x=h+a t^2$ and $y=k+2 a t$.
Position of a Point (x1, y1) with Respect to a Parabola y2 = 4ax
The point lies outside, on or inside the parabola according as
Parameteric Relation between the Coordinates of the Ends of a Focal Chord of a Parabola
Let be a parabola, if be a focal chord.
Then, and
Since, passes through the focus .
are collinear.
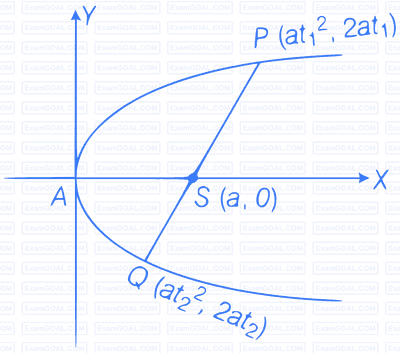
Slope of Slope of
or
or ,
Note :
If one extremity of a focal chord is then the other extremity becomes .
Then, and
Since, passes through the focus .
are collinear.

Slope of Slope of
or
or ,
Note :
If one extremity of a focal chord is then the other extremity becomes .
CHORD JOINING TWO POINTS
The equation of a chord of the parabola joining its two points and is
Note :
(i) If is focal chord then .
(ii) Extremities of focal chord can be taken as
(iii) If then chord always passes a fixed point .
Note :
(i) If is focal chord then .
(ii) Extremities of focal chord can be taken as
(iii) If then chord always passes a fixed point .
LINE AND A PARABOLA
(a) The line meets the parabola in two points real, coincident or imaginary according as a > = < cm.
condition of tangency is, .
Note : Line will be tangent to parabola
ay if .
(b) Length of the chord intercepted by the parabola ax on the line is :
.
Note : length of the focal chord making an angle with the -axis is .
condition of tangency is, .
Note : Line will be tangent to parabola
ay if .
(b) Length of the chord intercepted by the parabola ax on the line is :
.
Note : length of the focal chord making an angle with the -axis is .
LENGTH OF SUBTANGENT AND SUBNORMAL
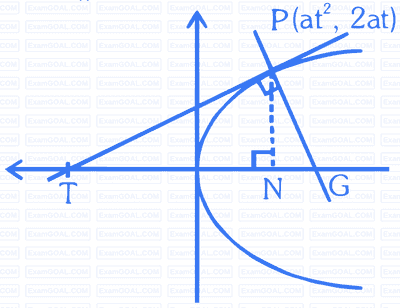
PT and PG are the tangent and normal respectively at the point to the parabola .
Then length of subtangent twice the abscissa of the point (Subtangent is always bisected by the vertex)
length of subnormal which is constant for all points on the parabola and equal to its semi latus rectum (2a).
TANGENT TO THE PARABOLA y2 = 4ax
(a) Point form :
Equation of tangent to the given parabola at its point is
(b) Slope form :
Equation of tangent to the given parabola whose slope is ' ', is
Point of contact is
(c) Parametric form :
Equation of tangent to the given parabola at its point , is
Note : Point of intersection of the tangents at the point is ]. (i.e. G.M. and A.M. of abscissae and ordinates of the points)
Equation of tangent to the given parabola at its point is
(b) Slope form :
Equation of tangent to the given parabola whose slope is ' ', is
Point of contact is
(c) Parametric form :
Equation of tangent to the given parabola at its point , is
Note : Point of intersection of the tangents at the point is ]. (i.e. G.M. and A.M. of abscissae and ordinates of the points)
NORMAL TO THE PARABOLA y2 = 4ax
(a) Point form : Equation of normal to the given parabola at its point
is
(b) Slope form : Equation of normal to the given parabola whose slope is ' ', is
foot of the normal is
(c) Parametric form :
Equation of normal to the given parabola at its point , is
Note :
(i) Point of intersection of normals at is .
(ii) If the normal to the parabola at the point , meets the parabola again at the point , then .
(iii) If the normals to the parabola at the points intersect again on the parabola at the point ' ' then and the line joining passes through a fixed point .
is
(b) Slope form : Equation of normal to the given parabola whose slope is ' ', is
foot of the normal is
(c) Parametric form :
Equation of normal to the given parabola at its point , is
Note :
(i) Point of intersection of normals at is .
(ii) If the normal to the parabola at the point , meets the parabola again at the point , then .
(iii) If the normals to the parabola at the points intersect again on the parabola at the point ' ' then and the line joining passes through a fixed point .
PAIR OF TANGENTS
The equation of the pair of tangents which can be drawn from any point outside the parabola to the parabola is given by : , where :
CHORD OF CONTACT
Equation of the chord of contact of tangents drawn from a point is
Remember that the area of the triangle formed by the tangents from the point and the chord of contact is . Also note that the chord of contact exists only if the point is not inside.
Remember that the area of the triangle formed by the tangents from the point and the chord of contact is . Also note that the chord of contact exists only if the point is not inside.
CHORD WITH A GIVEN MIDDLE POINT
Equation of the chord of the parabola $\mathrm{y}^2=4 \mathrm{ax}$ whose middle point is
$\left(x_1, y_1\right)$ is $y-y_1=\frac{2 a}{y_1}\left(x-x_1\right)$.
This reduced to $T=S_1$
where $T \equiv y y_1-2 a\left(x+x_1\right) \quad \& \quad S_1 \equiv y_1{ }^2-4 a x_1$.
$\left(x_1, y_1\right)$ is $y-y_1=\frac{2 a}{y_1}\left(x-x_1\right)$.
This reduced to $T=S_1$
where $T \equiv y y_1-2 a\left(x+x_1\right) \quad \& \quad S_1 \equiv y_1{ }^2-4 a x_1$.
DIAMETER
The locus of the middle points of a system of parallel chords of a Parabola is called a DIAMETER. Equation to the diameter of a parabola is , where slope of parallel chords.