STANDARD EQUATION AND DEFINITION
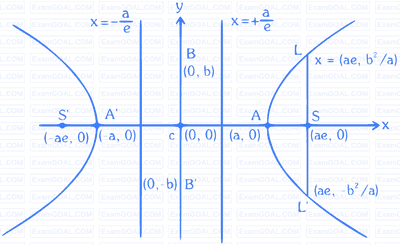
Standard equation of the hyperbola is ,
where
or
i.e.
(a) Foci :
(b) Equations of directrices :
and
(c) Vertices :
and
(d) Latus rectum :
(i) Equation:
(ii) Length (distance from focus to directrix)
(iii) Ends :
(e) (i) Transverse Axis : The line segment of length in which the foci both lie is called the Transverse Axis of the Hyperbola.
(ii) Conjugate Axis :
The line segment between the two points is called as the Conjugate Axis of the Hyperbola. The Transverse Axis \& the Conjugate Axis of the hyperbola are together called the Principal axis of the hyperbola.
(f) Focal Property :
The difference of the focal distances of any point on the hyperbola is constant and equal to transverse axis i.e. || . The distance focal length.
(g) Focal distance :
Distance of any point on hyperbola from foci
CONJUGATE HYPERBOLA
Two hyperbolas such that transverse and conjugate axis of one hyperbola are respectively the conjugate and the transverse axis of the other are called Conjugate Hyperbolas of each other. eg. and are conjugate hyperbolas of each other.
Note :
(i) If and are the eccentricities of the hyperbola \& its conjugate then .
(ii) The foci of a hyperbola and its conjugate are concyclic and form the vertices of a square.
(iii) Two hyperbolas are said to be similar if they have the same eccentricity.
Note :
(i) If and are the eccentricities of the hyperbola \& its conjugate then .
(ii) The foci of a hyperbola and its conjugate are concyclic and form the vertices of a square.
(iii) Two hyperbolas are said to be similar if they have the same eccentricity.
AUXILIARY CIRCLE

A circle drawn with centre and transverse axis as a diameter is called the Auxiliary Circle of the hyperbola. Equation of the auxiliary circle is .
Note from the figure that and are called the "Corresponding Points" on the hyperbola and the auxiliary circle. ' ' is called the eccentric angle of the point 'P' on the hyperbola. .
POSITION OF A POINT 'P' WITH RESPECT TO A HYPERBOLA
The quantity is positive, zero or negative according as the point lies within, upon or outside the curve.
LINE AND A HYPERBOLA
The straight line is a secant, a tangent or passes outside the hyperbola according as : c2 > = < a2m2 - b2.
Equation of a chord of the hyperbola joining its two points and is
Equation of a chord of the hyperbola joining its two points and is
TANGENT TO THE HYPERBOLA
(a) Point form : Equation of the tangent to the given hyperbola at the point is .
Note : In general two tangents can be drawn from an external point to the hyperbola and they are and , where and are roots of the equation . If , then no tangent can be drawn from to the hyperbola.
(b) Slope form : The equation of tangents of slope to the given hyperbola is . Point of contact are
Note that there are two parallel tangents having the same slope .
(c) Parametric form : Equation of the tangent to the given hyperbola at the point is
Note : Point of intersection of the tangents at and is
Note : In general two tangents can be drawn from an external point to the hyperbola and they are and , where and are roots of the equation . If , then no tangent can be drawn from to the hyperbola.
(b) Slope form : The equation of tangents of slope to the given hyperbola is . Point of contact are
Note that there are two parallel tangents having the same slope .
(c) Parametric form : Equation of the tangent to the given hyperbola at the point is
Note : Point of intersection of the tangents at and is
NORMAL TO THE HYPERBOLA
(a) Point form :
Equation of the normal to the given hyperbola at the point on it is .
(b) Slope form :
The equation of normal of slope to the given hyperbola is
foot of normal are
(c) Parametric form :
The equation of the normal at the point to the
given hyperbola is
Equation of the normal to the given hyperbola at the point on it is .
(b) Slope form :
The equation of normal of slope to the given hyperbola is
foot of normal are
(c) Parametric form :
The equation of the normal at the point to the
given hyperbola is
DIRECTOR CIRCLE
The locus of the intersection of tangents which are at right angles is known as the Director Circle of the hyperbola. The equation to the director circle is: .
If this circle is real; if the radius of the circle is zero \& it reduces to a point circle at the origin. In this case the centre is the only point from which the tangents at right angles can be drawn to the curve.
If , the radius of the circle is imaginary, so that there is no such circle \& so no tangents at right angle can be drawn to the curve.
If this circle is real; if the radius of the circle is zero \& it reduces to a point circle at the origin. In this case the centre is the only point from which the tangents at right angles can be drawn to the curve.
If , the radius of the circle is imaginary, so that there is no such circle \& so no tangents at right angle can be drawn to the curve.
CHORD OF CONTACT
If and be the tangents from point
to the Hyperbola , then the equation of the chord of
contact is or at .
to the Hyperbola , then the equation of the chord of
contact is or at .
PAIR OR TANGENTS
If be any point lies outside the Hyperbola and
a pair of tangents can be drawn to it from . Then the equation of pair of tangents of and is
where
i.e.
a pair of tangents can be drawn to it from . Then the equation of pair of tangents of and is
where
i.e.
EQUATION OF CHORD WITH MID POINT (x1, y1)
The equation of the chord of the ellipse ,
whose mid-point be is
where
i.e.
whose mid-point be is
where
i.e.
RECTANGULAR HYPERBOLA
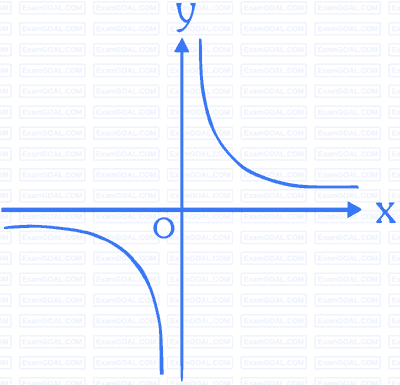
Rectangular hyperbola referred to its asymptotes as axis of coordinates.
(a) Equation is with parametric representation , .
(b) Equation of a chord joining the points and is with slope
(c) Equation of the tangent at is and at is .
(d) Equation of normal is
(e) Chord with a given middle point as is .