Standard equation of an ellipse referred to its principal axis along

the co-ordinate axis is $\frac{\mathbf{x}^2}{\mathbf{a}^2}+\frac{\mathbf{y}^2}{\mathbf{b}^2}=\mathbf{1}$. where $\mathrm{a}>\mathrm{b} $

and $ \mathrm{~b}^2=\mathrm{a}^2\left(1-e^2\right)$ $\Rightarrow \mathrm{a}^2-\mathrm{b}^2=\mathrm{a}^2 \mathrm{e}^2$. where $e=$ eccentricity $(0 < e < 1)$.


FOCI : S $\equiv(a e, 0) $ and $ S^{\prime} \equiv(-a e, 0)$

(a) Equation of directrices :

$ \mathrm{x}=\frac{\mathrm{a}}{\mathrm{e}} $ and $\mathrm{x}=-\frac{\mathrm{a}}{\mathrm{e}} \text {. } $

(b) Vertices :

$$ \mathrm{A}^{\prime} \equiv(-\mathrm{a}, 0) \quad \& \mathrm{~A} \equiv(\mathrm{a}, 0) . $$

(c) Major axis :

The line segment A'A in which the foci $\mathrm{S}^{\prime} \& \mathrm{~S}$ lie is of length $2 \mathrm{a} $ and is called the major axis $(\mathrm{a}>\mathrm{b})$ of the ellipse. Point of intersection of major axis with directrix is called the foot of the directrix (Z) $\left(\pm \frac{a}{e}, 0\right)$.

(d) Minor Axis : The y-axis intersects the ellipse in the points $B^{\prime} \equiv(0,-b) $and $B \equiv(0, b)$. The line segment $B^{\prime} B$ of length $2 b(b
(e) Principal Axis : The major \& minor axis together are called Principal Axis of the ellipse.

(f) Centre : The point which bisects every chord of the conic drawn through it is called the centre of the conic. $\mathrm{C} \equiv(0,0)$ the origin is the centre of the ellipse $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$

(g) Diameter : A chord of the conic which passes through the centre is called a diameter of the conic.

(h) Focal Chord : A chord which passes through a focus is called a focal chord.

(i) Double Ordinate : A chord perpendicular to the major axis is called a double ordinate with respect to major axis as diameter.

(j) Latus Rectum : The focal chord perpendicular to the major axis is called the latus rectum.

(i) Length of latus rectum :

$$ \left(L^{\prime}\right)=\frac{2 b^2}{a}=\frac{(\text { minor axis })^2}{\text { major axis }}=2 \mathrm{a}\left(1-e^2\right) $$

(ii) Equation of latus rectum : $x=\pm a e$.

(iii) Ends of the latus rectum are $\mathrm{L}\left(\mathrm{a}, \frac{\mathrm{b}^2}{\mathrm{a}}\right), \mathrm{L}^{\prime}\left(\mathrm{a},-\frac{\mathrm{b}^2}{\mathrm{a}}\right)$, $\mathrm{L}_1\left(-\mathrm{ae}, \frac{\mathrm{b}^2}{\mathrm{a}}\right)$ and $\mathrm{L}_1{ }^{\prime}\left(-\mathrm{ae},-\frac{\mathrm{b}^2}{\mathrm{a}}\right)$.

(k) Focal radii : $\mathrm{SP}=\mathrm{a}-e \mathrm{x}$ and $\mathrm{S} ' \mathrm{P}=\mathrm{a}+e \mathrm{x}$

$\Rightarrow \mathrm{SP}+\mathrm{S} ' \mathrm{P}=2 \mathrm{a}=$ Major axis.

(l) Eccentricity : $e=\sqrt{1-\frac{b^2}{a^2}}$

$$ \frac{x^2}{a^2}+\frac{y^2}{b^2}=1,(a < b) $$

(a) $\mathrm{AA}^{\prime}=$ Minor axis $=2 \mathrm{a}$

(b) $\mathrm{BB}^{\prime}=$ Major axis $=2 \mathrm{~b}$

(c) $\mathrm{a}^2=\mathrm{b}^2\left(1-\mathrm{e}^2\right)$

(d) Latus rectum

$$ \begin{aligned} &L^{\prime}=L_1 L_1^{\prime}=\frac{2 a^2}{b} . \\\\ &\text { equation } y=\pm b e \end{aligned} $$

(e) Ends of the latus rectum are:

$$ \mathrm{L}\left(\frac{\mathrm{a}^2}{\mathrm{~b}}, \mathrm{be}\right), \mathrm{L}^{\prime}\left(-\frac{\mathrm{a}^2}{\mathrm{~b}}, \mathrm{be}\right), \mathrm{L}_1\left(\frac{\mathrm{a}^2}{\mathrm{~b}},-\mathrm{be}\right), \mathrm{L}_1^{\prime} \left(-\frac{\mathrm{a}^2}{\mathrm{~b}},-\mathrm{be}\right) $$

(f) Equation of directrix $y=\pm \frac{b}{e}$.

(g) Eccentricity $: e=\sqrt{1-\frac{\mathrm{a}^2}{\mathrm{~b}^2}}$

The point $P\left(x_1, y_1\right)$ lies outside, inside or on the ellipse according as :

$$ \frac{x_1^2}{a^2}+\frac{y_1^2}{b^2}-1><\text { or }=0 $$

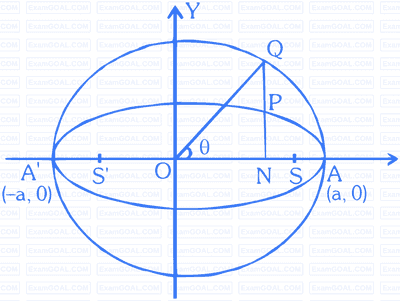
A circle described on major axis as diameter is called the auxiliary circle. Let $\mathrm{Q}$ be a point on the auxiliary circle $x^2+y^2=a^2$ such that QP produced is perpendicular to the $\mathrm{x}$-axis then $\mathrm{P}$ and $\mathrm{Q}$ are called as the CORRESPONDING POINTS on the ellipse and the auxiliary circle respectively. ' $\theta$ ' is called the ECCENTRIC ANGLE of the point $P$ on the ellipse $(0\le \theta <2\pi )$. Note that

$\frac{l(\mathrm{PN})}{l(\mathrm{QN})}=\frac{\mathrm{b}}{\mathrm{a}}=\frac{\text{ Semi minor axis }}{\text{ Semi major axis }}$

Hence "If from each point of a circle perpendiculars are drawn upon a fixed diameter then the locus of the points dividing these perpendiculars in a given ratio is an ellipse of which the given circle is the auxiliary circle".

The equations $\mathrm{x}=\mathrm{a}\cos \theta$ and $\mathrm{y}=\mathrm{b}\sin \theta$ together represent

the ellipse $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$ where $\theta$ is a parameter (eccentric angle).

Note that if $P(\theta )\equiv (a\cos \theta ,b\sin \theta )$ is on the ellipse then ; $Q(\theta )\equiv (a\cos \theta ,a\sin \theta )$ is on the auxiliary circle.

The line $y=mx+c$ meets the ellipse $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$ in two real points, coincident or imaginary according as $c^2$ is $<=$ or $>a^2{m}^2+b^2$.

Hence $y=mx+c$ is tangent to the ellipse $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$ if $c^2=a^2{m}^2+b^2$.

The equation to the chord of the ellipse joining two points with eccentric angles $\alpha \&\beta$ is given by

$\frac{x}{a}\cos \frac{\alpha +\beta }{2}+\frac{y}{b}\sin \frac{\alpha +\beta }{2}=\cos \frac{\alpha -\beta }{2}$.

(a) Point form :

Equation of tangent to the ellipse $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$ at its point $\left(x_1, y_1\right)$ is $\frac{x x_1}{a^2}+\frac{y y_1}{b^2}=1$

(b) Slope form :

Equation of tangent to the ellipse $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$ whose slope is ' $m$ ', is $y=m x \pm \sqrt{a^2 m^2+b^2}$

Point of contact are $\left(\frac{\pm a^2 m}{\sqrt{a^2 m^2+b^2}}, \frac{\mp b^2}{\sqrt{a^2 m^2+b^2}}\right)$

(c) Parametric form :

Equation of tangent to the given ellipse at its point $(a \cos \theta, b \sin \theta)$, is $\frac{x \cos \theta}{a}+\frac{y \sin \theta}{b}=1$

(a) Point form : Equation of the normal to the given ellipse at $\left(x_1,y_1\right)$ is

$\frac{a^{2}x}{x_1}-\frac{b^{2}y}{y_1}=a^2-b^2=a^2{e}^2$.

(b) Slope form : Equation of a normal to the given ellipse whose slope is ' $m$ ' is $y=mx\mp \frac{\left(a^2-b^2\right)m}{\sqrt{a^2+b^2{m}^2}}$.

(c) Parametric form : Equation of the normal to the given ellipse at the point $(a\cos \theta ,b\sin \theta )$ is $ax\sec \theta -by\mathrm{cosec}\theta =\left(a^2-b^2\right)$.

If $\mathrm{PA}$ and $\mathrm{PB}$ be the tangents from point $\mathrm{P}\left({\mathrm{x}}_1,{\mathrm{y}}_1\right)$ to the ellipse $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$

then the equation of the chord of contact $\mathrm{AB}$ is $\frac{{\mathrm{xx}}_1}{{\mathrm{a}}^2}+\frac{\mathrm{y}{\mathrm{y}}_1}{{\mathrm{b}}^2}=1$ or $\mathrm{T}=0$ at $\left({\mathrm{x}}_1,{\mathrm{y}}_1\right)$

If $\mathrm{P}\left({\mathrm{x}}_1,{\mathrm{y}}_1\right)$ be any point lies outside the ellipse $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$ and a pair of tangents PA, PB can be drawn to it from $P$.

Then the equation of pair of tangents of $\mathrm{PA}$ and $\mathrm{PB}$ is ${\mathrm{SS}}_1={\mathrm{T}}^2$

where ${\mathrm{S}}_1=\frac{{\mathrm{x}}_1^2}{{\mathrm{a}}^2}+\frac{y_1^2}{b^2}-1,\mathrm{T}=\frac{{\mathrm{xx}}_1}{{\mathrm{a}}^2}+\frac{{\mathrm{yy}}_1}{{\mathrm{b}}^2}-1$

i.e. $\left(\frac{x^2}{a^2}+\frac{y^2}{b^2}-1\right)\left(\frac{x_1^2}{a^2}+\frac{y_1^2}{b^2}-1\right)={\left(\frac{x{x}_1}{a^2}+\frac{y{y}_1}{b^2}-1\right)}^2$

Locus of the point of intersection of the tangents which meet at right angles is called the Director Circle. The equation to this locus is $x^2+y^2=a^2+b^2$ i.e. a circle whose centre is the centre of the ellipse and whose radius is the length of the line joining the ends of the major and minor axis.

The equation of the chord of the ellipse $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$,

whose mid-point be $\left({\mathrm{x}}_1,{\mathrm{y}}_1\right)$ is $\mathrm{T}={\mathrm{S}}_1$

where $T=\frac{x{x}_1}{a^2}+\frac{y{y}_1}{b^2}-1,S_1=\frac{x_1^2}{a^2}+\frac{y_1^2}{b^2}-1$

i.e. $\left(\frac{x_1}{a^2}+\frac{y{y}_1}{b^2}-1\right)=\left(\frac{x_1^2}{a^2}+\frac{y_1^2}{b^2}-1\right)$