Before the gun is fired, both the gun and the bullet are at rest. Therefore, the total momentum of the gun-bullet system is zero. After the gun is fired, the bullet moves forward and the gun recoils backwards. Let $m_{b}$ and $m_{g}$ be the masses of the bullet and the gun. If $\mathbf{v}_{b}$ and $\mathbf{v}_{g}$ are their respective velocities after firing, the total momentum of the gun-bullet system after firing is $\left(m_{b} \mathbf{v}_{b}+m_{g} \mathbf{v}_{g}\right)$. From the law of conservation of momentum, the total momentum after and before the gun is fired must be the same, i.e.

$$ \begin{aligned} &m_{b} \mathbf{v}_{b}+m_{g} \mathbf{v}_{g}=0 \\\\ &\text { or } \quad \mathbf{v}_{g}=-\frac{m_{b} \mathbf{v}_{b}}{m_{g}} \\\\ &\text { The negative sign indicates that the gun recoils in a } \\ &\text { direction opposite to that of the bullet. In terms of mag- } \\ &\text { nitudes, we have } \end{aligned} $$

$$ v_{g}=\frac{m_{b} v_{b}}{m_{g}} $$

Impulse of force is defined as average force multiplied with time interval for which it acts on an object.

$\vec{I}=\vec{F} \Delta t=\vec{p}_2-\vec{p}_1$

Let us consider a case of $n$-particle system and total linear momentum of the system can be written as follows :

$$ \vec{P}=m_1 \vec{v}_1+m_2 \vec{v}_2+\ldots .+m_{\mathrm{n}} \vec{v}_{\mathrm{n}} $$

If $\vec{V}_{\mathrm{cm}}$ is the velocity of centre of mass of the system and $M$ is the total mass of the system then

$$ M \vec{V}_{\mathrm{cm}}=m_1 \vec{v}_1+m_2 \vec{v}_2+\ldots . .+m_{\mathrm{n}} \vec{v}_{\mathrm{n}} $$

Hence, the total linear momentum of the system can be written as follows :

$$ \vec{P}=M \vec{V}_{\mathrm{cm}} $$

On differentiating above equation we get the following relation :

$$ \begin{aligned} & \frac{d \vec{P}}{d t}=M \frac{d \vec{V}_{\mathrm{cm}}}{d t} \\\\ \Rightarrow \quad & \frac{d \vec{P}}{d t}=M \vec{a}_{\mathrm{cm}} \end{aligned} $$

We know that $\vec{F}_{\text {ext }}=M \vec{a}_{\mathrm{cm}}$;

hence above equation can be written as follows :

$$ \frac{d \vec{P}}{d t}=\vec{F}_{\text {ext }} $$

If $\vec{F}_{\text {ext }}=0 \Rightarrow $ $ \frac{d \vec{P}}{d t}=0 \Rightarrow \vec{P}=$ constant.

Thus, if no net external force acts on a system, the total linear momentum of the system remains constant; the total linear momentum being the vector sum of linear momentum of individual particles, i.e.

$$ \vec{P}=\vec{P}_1+\vec{P}_2+\cdots+\vec{P}_n $$

Let $m_1, m_2, m_3, \ldots, m_{\mathrm{n}}$ be the masses of $n$ particles constituting a system. Assume that these particles are distributed in three-dimensional space and the coordinates of these particles are $\left(x_1, y_1, z_1\right),\left(x_2, y_2, z_2\right),\left(x_3, y_3, z_3\right), \ldots \ldots\left(x_n, y_n, z_n\right)$, respectively. The location of centre of mass of the given system is mass-based average position and can be written as follows:

$$ X_{\mathrm{cm}}=\frac{m_1 x_1+m_2 x_2+m_3 x_3+\ldots+m_{\mathrm{n}} x_{\mathrm{n}}}{m_1+m_2+m_3+\ldots . .+m_{\mathrm{n}}}=\frac{\sum\limits_{i=1}^n m_{\mathrm{i}} x_{\mathrm{i}}}{\sum\limits_{i=1}^n m_{\mathrm{i}}} $$

$Y_{\mathrm{cm}}=\frac{m_1 y_1+m_2 y_2+m_3 y_3+\ldots+m_{\mathrm{n}} y_{\mathrm{n}}}{m_1+m_2+m_3+\ldots . .+m_{\mathrm{n}}}=\frac{\sum\limits_{i=1}^n m_{\mathrm{i}} y_{\mathrm{i}}}{\sum\limits_{i=1}^n m_{\mathrm{i}}}$

$Z_{\mathrm{cm}}=\frac{m_1 z_1+m_2 z_2+m_3 z_3+\ldots+m_{\mathrm{n}} z_{\mathrm{n}}}{m_1+m_2+m_3+\ldots . .+m_{\mathrm{n}}}=\frac{\sum\limits_{i=1}^n m_{\mathrm{i}} z_{\mathrm{i}}}{\sum\limits_{i=1}^n m_{\mathrm{i}}}$

If ${\mathbf{r}}_1,{\mathbf{r}}_2,\dots {\mathbf{r}}_n$ are the position vectors of masses $m_1,m_2,\dots$ $m_n$, respectively, thle position vector of the centre of mass of the system of particles is given by

${\mathbf{r}}_{\mathrm{CM}}=\frac{m_1{\mathbf{r}}_1+m_2{\mathbf{r}}_2+\cdots +m_n{\mathbf{r}}_n}{m_1+m_2+\cdots +m_n}$

The velocity of the centre of mass is given by

$\begin{array}{rl}{\mathbf{v}}_{\mathrm{CM}}& =\frac{d{\mathbf{r}}_{CM}}{dt}\\ & \\ & =\frac{m_1\frac{d{\mathbf{r}}_1}{dt}+m_2\frac{d{\mathbf{r}}_2}{dt}+\dots +m_n\frac{d{\mathbf{r}}_n}{dt}}{m_1+m_2+\dots +m_n}\\ & \\ {\mathbf{v}}_{\mathrm{CM}}& =\frac{m_1{\mathbf{v}}_1+m_2{\mathbf{v}}_2+\dots +m_n{\mathbf{v}}_n}{m_1+m_2+\dots +m_n}\end{array}$

The acceleration of the centre of mass is given by

$\begin{array}{rl}{a}_{\mathrm{CM}}& =\frac{d{\mathbf{v}}_{\mathrm{CM}}}{dt}\\ & \\ & =\frac{m_1\frac{d{\mathbf{v}}_1}{dt}+m_2\frac{d{\mathbf{v}}_2}{dt}+\cdots +m_n\frac{d{\mathbf{v}}_n}{dt}}{m_1+m_2+\cdots +m_n}\end{array}$

$\Rightarrow {\mathbf{a}}_{\mathrm{CM}}=\frac{m_1{\mathbf{a}}_1+m_2{\mathbf{a}}_2+\cdots +m_n{\mathbf{a}}_n}{m_1+m_2+\cdots +m_n}$

${\mathbf{a}}_{\mathrm{CM}}=\frac{{\mathbf{F}}_1+{\mathbf{F}}_2+\cdots +{\mathbf{F}}_n}{m_1+m_2+\cdots +m_n}=\frac{\sum {\mathbf{F}}_{\mathrm{ext}}}{M}$

If $\sum {\mathrm{F}}_{\mathrm{ext}}=0$, then ${\mathrm{a}}_{\mathrm{CM}}=0$, i.e ${\mathrm{v}}_{\mathrm{CM}}=$ constant. Hence if no net external force acts on a system, its centre of mass will remain at rest or will move with a constant velocity.

(i) $\quad \vec{r}_{\text {COM }}=\frac{m_1 \vec{r}_1-m_2 \vec{r}_2}{m_1-m_2}$ or $\vec{r}_{\text {COM }}=\frac{A_1 \vec{r}_1-A_2 \vec{r}_2}{A_1-A_2}$

(ii) $\mathrm{x}_{\operatorname{com}}=\frac{\mathrm{m}_1 \mathrm{x}_1-\mathrm{m}_2 \mathrm{x}_2}{\mathrm{~m}_1-\mathrm{m}_2}$ or $\mathrm{x}_{\operatorname{com}}=\frac{\mathrm{A}_1 \mathrm{x}_1-\mathrm{A}_2 \mathrm{x}_2}{\mathrm{~A}_1-\mathrm{A}_2}$

$y_{\mathrm{COM}}=\frac{m_1 y_1-m_2 y_2}{m_1-m_2}$ or $y_{C O M}=\frac{A_1 y_1-A_2 y_2}{A_1-A_2}$ and

$$ \mathrm{z}_{\mathrm{COM}}=\frac{\mathrm{m}_1 \mathrm{z}_1-\mathrm{m}_2 \mathrm{z}_2}{\mathrm{~m}_1-\mathrm{m}_2} \text { or } \mathrm{z}_{\mathrm{COM}}=\frac{\mathrm{A}_1 \mathrm{z}_1-\mathrm{A}_2 \mathrm{z}_2}{\mathrm{~A}_1-\mathrm{A}_2} $$

Here, $m_1, A_1, \vec{r}_1, x_1, y_1$ and $z_1$ are the values for the whole mass while $m_2, A_2, \vec{r}_2, {x}_2, y_2$ and $z_2$ are the values for the mass which has been removed. Let us see two examples in support of the above theory.

$y_{\mathrm{cm}}=\frac{\mathrm{R}}{2}$

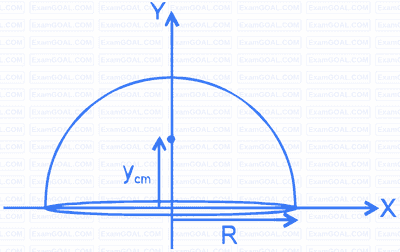
$\mathrm{y}_{\mathrm{cm}}=\frac{3 \mathrm{R}}{8}$

$\mathrm{y}_{\mathrm{cm}}=\frac{4 \mathrm{R}}{3 \pi}$

$\mathrm{y}_{\mathrm{cm}}=\frac{2 \mathrm{R}}{\pi}$

$$ x_{com}=\frac{\int_0^L x d m}{\int d m}=\frac{\int_0^L(x)\left(\frac{M}{L} d x\right)}{M}=\frac{1}{L} \int_0^L x d x=\frac{L}{2} $$

The $y$-coordinate of COM is $y$_com $=\frac{\int y d m}{\int d m}=0$.

Similarly, $z_{c o m}=0$

i.e., the coordinates of $\mathrm{COM}$ of the rod are $\left(\frac{\mathrm{L}}{2}, 0,0\right)$, i.e. it lies at the center of the rod.

For continuous mass distribution the center of mass can be located by replacing summation sign with an integral sign. Proper limits for the integral are chosen according to the situation

$x_{c m}=\frac{\int x d m}{\int d m}, $

$y_{c m}=\frac{\int y d m}{\int d m}, $

$z_{c m}=\frac{\int z d m}{\int d m}$

Note: If an object has symmetric mass distribution about $\mathrm{x}$ axis then $\mathrm{y}$ coordinate of COM is zero and vice-versa.

X-coordinate of the centre of mass can be written as follows :

$$ X_{\mathrm{cm}}=\frac{m_1 x_1+m_2 x_2}{m_1+m_2}=\frac{m_1(0)+m_2(d)}{m_1+m_2}=\frac{m_2 d}{m_1+m_2} $$

Note :

1. We know that the centre of mass of a two-particle system lies on the line joining the particles.

2. The distance of the centre of mass from particle $m_1$ is $\frac{m_2 d}{m_1+m_2}$ and so the distance of centre of mass from $m_2$ will be $d-\frac{m_2 d}{m_1+m_2}=\frac{m_1 d}{m_1+m_2}$