Horizontal Projectile
Consider a point P x( , y) at time t.

Distance Travelled in Horizontal Motion (HM) :
Since acceleration due to gravity acts along the vertical and hence, has got no component along the horizontal i.e., $a_x=0$.
So, horizontal motion is a non-accelerated motion with uniform velocity.
$$ \Rightarrow \quad x=u_x t=u t $$
Distance Travelled in Vertical Motion (VM)
$a_y=g$ and $u_y=0$
$$ \begin{aligned} &\Rightarrow y=\frac{1}{2} g t^2 \end{aligned} $$
Equation of Trajectory :
$$ y=\left(\frac{g}{2 u^2}\right) x^2 $$
which is the equation of a parabola.
Velocity at Any Instant ( $t$ ) in Horizontal Motion (HM) :
Since horizontal motion is non-accelerated,
$$ \Rightarrow v_x=u_x=u $$
Velocity at Any Instant ( $t$ ) in Vertical Motion (VM) :
$$ \begin{gathered} \Rightarrow \quad|\vec{v}|=v=\sqrt{u^2+g^2 t^2} \end{gathered} $$
If $\beta$ is the angle made by $\vec{v}$ with the horizontal, then
$$ \tan \beta=\frac{v_y}{v_x}=\frac{g t}{u} $$
Time of Flight (T) :
If $h$ is the distance of the ground from the point of launch, $T$ is the time taken to strike the ground, then
$$ \begin{aligned} h &=\frac{1}{2} g T^2 \\ \Rightarrow T &=\sqrt{\frac{2 h}{g}} \end{aligned} $$
Horizontal Range (R) :
$R$ is the range of the projectile when it hits the ground, then
$$ \begin{aligned} R=u T \\ \Rightarrow R &=u \sqrt{\frac{2 h}{g}} \end{aligned} $$

Distance Travelled in Horizontal Motion (HM) :
Since acceleration due to gravity acts along the vertical and hence, has got no component along the horizontal i.e., $a_x=0$.
So, horizontal motion is a non-accelerated motion with uniform velocity.
$$ \Rightarrow \quad x=u_x t=u t $$
Distance Travelled in Vertical Motion (VM)
$a_y=g$ and $u_y=0$
$$ \begin{aligned} &\Rightarrow y=\frac{1}{2} g t^2 \end{aligned} $$
Equation of Trajectory :
$$ y=\left(\frac{g}{2 u^2}\right) x^2 $$
which is the equation of a parabola.
Velocity at Any Instant ( $t$ ) in Horizontal Motion (HM) :
Since horizontal motion is non-accelerated,
$$ \Rightarrow v_x=u_x=u $$
Velocity at Any Instant ( $t$ ) in Vertical Motion (VM) :
$$ \begin{gathered} \Rightarrow \quad|\vec{v}|=v=\sqrt{u^2+g^2 t^2} \end{gathered} $$
If $\beta$ is the angle made by $\vec{v}$ with the horizontal, then
$$ \tan \beta=\frac{v_y}{v_x}=\frac{g t}{u} $$
Time of Flight (T) :
If $h$ is the distance of the ground from the point of launch, $T$ is the time taken to strike the ground, then
$$ \begin{aligned} h &=\frac{1}{2} g T^2 \\ \Rightarrow T &=\sqrt{\frac{2 h}{g}} \end{aligned} $$
Horizontal Range (R) :
$R$ is the range of the projectile when it hits the ground, then
$$ \begin{aligned} R=u T \\ \Rightarrow R &=u \sqrt{\frac{2 h}{g}} \end{aligned} $$
OBLIQUE PROJECTILE
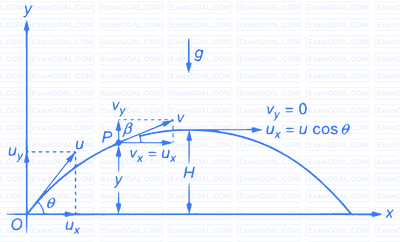
Trajectory: $y=x \tan \theta-\frac{g x^2}{2 u^2 \cos ^2 \theta}$
Time of flight: $T=\frac{2 u \sin \theta}{g}$
Maximum height: $H=\frac{u^2 \sin ^2 \theta}{2 g}$
Horizontal range: $R=\frac{u^2 \sin 2 \theta}{g}$
Different Situation in Projectile Motion
Case-1: Particle projected at an angle q above the
horizontal

The situation is shown in figure. In this case, we have
$$ y=-h, u_x=u \cos \theta, u_y=u \sin \theta \text { and } a_y=-g $$
Case-2 : Particle projected at an angle q below the horizontal

The situation is shown in figure. In this case, we have
$$ y=+h, u_x=u \cos \theta, u_y=+u \sin \theta \text { and } a_y=+g $$

The situation is shown in figure. In this case, we have
$$ y=-h, u_x=u \cos \theta, u_y=u \sin \theta \text { and } a_y=-g $$
Case-2 : Particle projected at an angle q below the horizontal

The situation is shown in figure. In this case, we have
$$ y=+h, u_x=u \cos \theta, u_y=+u \sin \theta \text { and } a_y=+g $$
Relative Motion Between Two Projectiles/Motion of One Projectile as Seen from Another Projectile
Suppose that two particles are projected from the ground with speeds $u_1$ and $u_2$ at angles $\alpha_1$ and $\alpha_2$ as shown in figure. Acceleration of both the particles is $g$ downwards. So, relative acceleration between them is zero because
$$ a_{12}=a_1-a_2=g-g=\text { zero } $$

i.e., the relative motion between the two particles is uniform. Now,
$$ \begin{aligned} &\left(u_1\right)_x=u_1 \cos \alpha_1 \text { and }\left(u_2\right)_x=u_2 \cos \alpha_2 \\\\ &\left(u_1\right)_y=u_1 \sin \alpha_1 \text { and }\left(u_2\right)_y=u_2 \sin \alpha_2 \end{aligned} $$
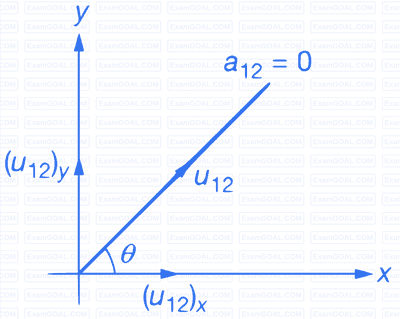
Therefore,
$$ \left(u_{12}\right)_x=\left(u_1\right)_x-\left(u_2\right)_x=u_1 \cos \alpha_1-u_2 \cos \alpha_2 $$
and $\left(u_{12}\right)_y=\left(u_1\right)_y-\left(u_2\right)_y=u_1 \sin \alpha_1-u_2 \sin \alpha_2$
$\left(u_{12}\right)_x$ and $\left(u_{12}\right)_y$ are the $x$ and $y$ components of relative velocity of 1 with respect to 2 .
And $\theta=\tan ^{-1}\left[\frac{\left(u_{12}\right)_y}{\left(u_{12}\right)_x}\right]$ with positive $x$-axis.
$$ a_{12}=a_1-a_2=g-g=\text { zero } $$

i.e., the relative motion between the two particles is uniform. Now,
$$ \begin{aligned} &\left(u_1\right)_x=u_1 \cos \alpha_1 \text { and }\left(u_2\right)_x=u_2 \cos \alpha_2 \\\\ &\left(u_1\right)_y=u_1 \sin \alpha_1 \text { and }\left(u_2\right)_y=u_2 \sin \alpha_2 \end{aligned} $$

Therefore,
$$ \left(u_{12}\right)_x=\left(u_1\right)_x-\left(u_2\right)_x=u_1 \cos \alpha_1-u_2 \cos \alpha_2 $$
and $\left(u_{12}\right)_y=\left(u_1\right)_y-\left(u_2\right)_y=u_1 \sin \alpha_1-u_2 \sin \alpha_2$
$\left(u_{12}\right)_x$ and $\left(u_{12}\right)_y$ are the $x$ and $y$ components of relative velocity of 1 with respect to 2 .
And $\theta=\tan ^{-1}\left[\frac{\left(u_{12}\right)_y}{\left(u_{12}\right)_x}\right]$ with positive $x$-axis.