Energy and Momentum of Photon
Energy $E$ and frequency $v$ of an electromagnetic radiation are related by the Planck's equation that is
$$ E=h v $$
$h \equiv$ Planck's constant $=6.63 \times 10^{-34} \mathrm{~J} \cdot \mathrm{s}$
For a photon moving in specific direction with velocity $c$, the energy $E$ and the momentum $p$ are related by the equation
$$ E=p c $$
$$ E=h v $$
$h \equiv$ Planck's constant $=6.63 \times 10^{-34} \mathrm{~J} \cdot \mathrm{s}$
For a photon moving in specific direction with velocity $c$, the energy $E$ and the momentum $p$ are related by the equation
$$ E=p c $$
Einstein's Photoelectric Equation
Maximum kinetic energy $K_{\max }$ of emitted photoelectrons equals the difference between energy $E$ of incident photon and work function $\phi$ of the metal.
$$ K_{\max }=E-\phi $$De Broglie hypothesis
Wavelength $\lambda$ assigned to a particle of mass $m$ moving with velocity $v$ is
$$ \lambda=\frac{h}{m v} $$
$$ \lambda=\frac{h}{m v} $$
Power of Source Emiting Photons
Power of the source is $P$ watt, we can say that the source is emitting light energy $P$ joule per second in the form of photons.
Number of Photons Emitted Per Seconds
If it is $100 \%$ efficient, then the number of photons emitted per second by the source can be given as
$$ n=\frac{\text { power of the source }}{\text { energy of a photon }}=\frac{P}{E}=\frac{P \lambda}{h c} $$
Note : These photons are assumed to be emitted uniformly in all directions. Here, we can consider that all the light energy emitted by the source is uniformly distributed in the spherical region with center at the source.
$$ n=\frac{\text { power of the source }}{\text { energy of a photon }}=\frac{P}{E}=\frac{P \lambda}{h c} $$
Note : These photons are assumed to be emitted uniformly in all directions. Here, we can consider that all the light energy emitted by the source is uniformly distributed in the spherical region with center at the source.
Intensity of Light
1. Due to beam of light :
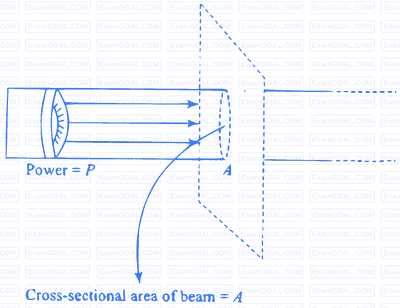
If a source of light emits a uniform cylindrical light beam of cross-sectional area $A$. If the source emits a total power P in the beam then intensity of light,
$I=\frac{P}{A} \mathrm{~W} \mathrm{~m}^{-2}$
1. Due to point source :
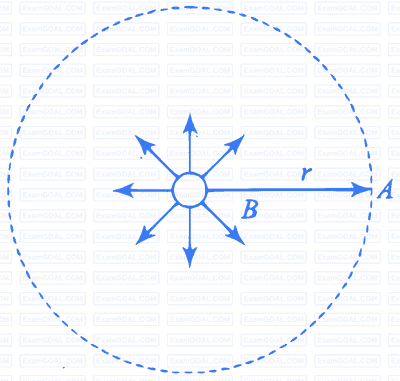
Intensity of light at a point $A$ and as well as all the point one circle, at a distance $r$ from the source, given as
$$ I=\frac{P}{4 \pi r^2} \mathrm{~W} \mathrm{~m}^{-2} $$
If a source of light emits a uniform cylindrical light beam of cross-sectional area $A$. If the source emits a total power P in the beam then intensity of light,
$I=\frac{P}{A} \mathrm{~W} \mathrm{~m}^{-2}$

1. Due to point source :

Intensity of light at a point $A$ and as well as all the point one circle, at a distance $r$ from the source, given as
$$ I=\frac{P}{4 \pi r^2} \mathrm{~W} \mathrm{~m}^{-2} $$