Volume of liquid or solid is volume occupied by liquid or solid.

Units of Volume

$ (i) m^3 $ (S.I Unit)

$ (ii) \mathrm{Cc} $ or $\mathrm{cm}^3$

$ (iii) \text { Liter }$

$ (iv) \mathrm{ML} $

Note :

(a) 1 Cc or cm³ $=\left(10^{-2}\right)^3 \mathrm{~m}^3=10^{-6} \mathrm{~m}^3 $

(b) 1 m³ = 1000 liter

(c) 1 liter = 1000 mL

(d) 1 cm³ = 1 mL

Relative Density of material-2 w.r.t material-1

= density of material-2 with respect to material-1

$=\frac{\text { density-2 }}{\text { density-1 }}=\frac{\rho_2}{\rho_1}$

Normally we take water as standard material to calculate relative density.

Water density = 1000 kg/m³ = 1 gm/cm³

Relative density of any material with respect to water is called as Specific Gravity(S.G) of material.

$$ \therefore $$ Specific Gravity(S.G) = $${{Density\,of\,Material} \over {Density\,of\,Water}}$$

Pressure is defined only for liquid and gases.

Pressure(P) = $${{Force\,Perpendicular\,to\,the\,Surface} \over {Surface\,Area}}$$

Note : Atmosphere pressure at the surface of the earth = 1 atm $=1.01 \times 10^5 \mathrm{~N} / \mathrm{m}^2=1.01 \times 10^5 \mathrm{~Pa}=76 \mathrm{~cm}$ (or $760 \mathrm{~mm}$ ) of column of mercury

Force = Pressure $$ \times $$ Area

$$ \Rightarrow $$ F = PA or F = $$\int {PdA} $$

Note :

(1) Force is prependicular to the surface.

(2) Force is away from the fluid means push force.

Pressure is equal at all points on the same horizontal plane inside a static fluid :

Let two points A and B lie on the same horizontal plane of a stationary fluid. A very narrow rod of cross-section S is drawn through the axis AB. Fluid is in equilibrium inside it.

If the pressures at points A and B are P₁ and P₂, the inward horizontal forces of cylinder are P₁$$\Delta$$S and P ₂$$\Delta$$S .

$$\therefore$$ Condition of equilibrium, P₁$$\Delta$$S = P₂$$\Delta$$S

$$\therefore$$ P₁ = P₂

The difference of pressure between two points separated by a depth h :

(P₂ –P₁ ) = h$$\rho $$g

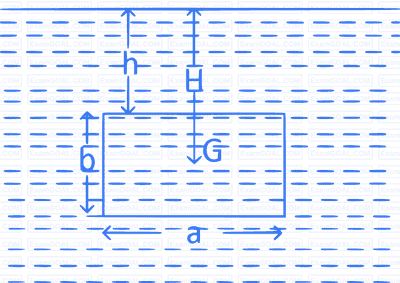
Let a rectangular plate be immersed vertically in a liquid of density $$\rho$$. Let width is a and height is b of the plate. The pressure at the surface of the plate is the depth h from the free surface of the liquid $${P_1} = h\rho g$$

$$\therefore$$ The depth of the lower edge of the plate $$(h + b)$$

$$\therefore$$ Pressure at the lower end of the plate $${P_2} = (h + b)\rho g$$

Since the pressure is proportional to the depth and the width is the same throughout the plate, in this case the average pressure is $$P = {{{P_1} + {P_2}} \over 2} = {{h\rho g + (h + b)\rho g} \over 2}$$

$$\therefore$$ $$P = \left( {h + {b \over 2}} \right)\rho g$$

The geometric center of mass of the plate is located at the intersection of the two diagonals, which is $${b \over 2}$$ below the top edge.

$$\therefore$$ Depth of center of gravity from free surface of liquid $$H = (h + b/2)$$

$$\therefore$$ Applied pressure at the geometric center of gravity $$ = H\rho g = (h + b/2)\rho g$$

$$ \therefore $$ Hence the average pressure exerted by the fluid on the plate is equal to the pressure exerted at its geometric center of gravity.

This principle applies to any size object, even if it is positioned diagonally.

Total thrust on the plate = Average pressure $$\times$$ Area

$$ = \left( {h + {b \over 2}} \right)\rho g \times ab$$

A body wholly or partially submerged in a fluid is buoyed up by a force equal to the weight of the displaced fluid.

This result known as Archimedes 'principle.

Thus, the magnitude of buoyant force (B) is given by, B = V_i$$\rho $$_Lg

Here, V_i = immersed volume of solid

$$\rho $$_L = density of liquid

and g = acceleration due to gravity

Consider an object of volume V and density $$\rho $$_s floating in a liquid of density $$\rho $$_L. Let V_i be the volume of object immersed in the liquid.

For equilibrium of object,

Weight = Upthrust

$$ \therefore $$ V$$\rho $$_sg = V_i$$\rho $$_Lg

$ \therefore \quad \frac{\mathrm{V}_{\mathrm{i}}}{\mathrm{V}}=\frac{\rho_{\mathrm{s}}}{\rho_{\mathrm{L}}}$

This is the fraction of volume immersed in liquid.

$$ \text { Percentage of volume immersed in liquid }=\frac{\mathrm{V}_{\mathrm{i}}}{\mathrm{V}} \times 100=\frac{\rho_{\mathrm{s}}}{\rho_{\mathrm{L}}} \times 100 $$

Note :

Three possibilties may now arise.

(i) If $$\rho $$_s < $$\rho $$_L, only fraction of body will be immersed in the liquid. This fraction will be given by the above equation.

(ii) If $$\rho $$_s = $$\rho $$_L, the whole of the rigid body will be immersed in the liquid. Hence the body remains floating in the liquid wherever it is left .

(iii) If $$\rho $$_s > $$\rho $$_L, the body will sink .

Let an object of volume V have density d.

$$\therefore$$ is the actual weight of the object W = Vdg

The object is fully immersed in a fluid of density $$\rho$$ ($$\rho$$ < d). The buoyancy exerted by the fluid on an object is equal to the weight of the fluid displaced by the object.

$$\therefore$$ Buoyancy $$B = V\rho g$$

As weight is downward and buoyancy is upward, their resultant $$W - B$$

This resultant force is the apparent weight of the object.

$$\therefore$$ The apparent weight of the object,

$$W' = W - B$$

$$ = vdg - v\rho g$$

$$\therefore$$ $$W' = v(d - \rho )g$$

Also $$W = vdg$$

$$\therefore$$ $$vg = {W \over d}$$

$$\therefore$$ $$W' = {W \over d}(d - \rho )$$

or, $$W' = W(1 - {\rho \over d})$$

The formula applies only to objects fully immersed in fluid.

Let a object has the density d and volume v.

$$\therefore$$ its weight, W = vdg

Buoyancy if the object is fully immersed in a liquid of density $$\rho$$

B = v$$\rho$$g

Case I : Objects are denser than liquids

If d > $$\rho$$ then W > B . So the force on the object will be downward. Let the downward acceleration of the object be a.

ma = W $$-$$ B

or, vda = vdg $$-$$ v$$\rho$$g

or, a = g(1 $$-$$ $$\rho$$/d)

If the object descends to a height H at time t from rest

$$H = {1 \over 2}a{t^2}$$

or, $$t = \sqrt {{{2H} \over a}} = \sqrt {{{2H} \over {g(1 - \rho /d)}}} $$

or, $$t = \sqrt {{{2Hd} \over {g(d - \rho )}}} $$

Case II : Density of object is less than that of liquid

If $$d < p$$ then $$W < B$$. So the force on the object will be upward. If the upward acceleration of the object is a

$$ma = B - W$$

or, $$vda = v\rho g - vdg$$

or, $$a = g\left( {{\rho \over d} - 1} \right)$$

If the object rises to a height H at time t from rest

$$t = \sqrt {{{2H} \over a}} = \sqrt {{{2H} \over {g\left( {{\rho \over d} - 1} \right)}}} $$

$$\therefore$$ $$t = \sqrt {{{2Hd} \over {g(\rho - d)}}} $$

(1) Pressure at point A, P_A = P₀ (air-liquid boundary)

(2) Pressure at point B, P_B = P_A + $$\rho$$gh

(3) Pressure at point D, P_D = P_B = P_A + $$\rho$$gh

(4) Pressure at point C, P_C = P_B + $$\rho$$gh₁ = P_A + $$\rho$$gH

(5) Force on bottom due to liquid = (P₀ + $$\rho$$gH) $$\times$$ A

where A = Area of the bottom surface

Here container is moving upward with acceleration a with liquid of density $$\rho$$.

Let pressure at point A = P_A

and pressure at point B = P_B

$$\therefore$$ P_B $$-$$ P_A = $$\rho$$(g + a)h

Here container is moving downward with acceleration a with liquid of density $$\rho$$.

Let pressure at point A = P_A

and pressure at point B = P_B

$$\therefore$$ P_B $$-$$ P_A = $$\rho$$(g $$-$$ a)h

In case of free fall acceleration a = g.

$$\therefore$$ P_B $$-$$ P_A = 0

Here container is moving horizontally with acceleration a with liquid of density $$\rho$$.

Free body diagram of liquid,

$$\therefore$$ P_BA $$-$$ P_AA = ma

$$\Rightarrow$$ P_BA $$-$$ P_AA = ($$\rho$$$$l$$A) a [$$\because$$ m = $$\rho$$A$$l$$ ]

$$\Rightarrow$$ P_B $$-$$ P_A = $$\rho$$a$$l$$

$$\therefore$$ Pressure at B is more than pressure at A. So, we can say pressure increases opposite direction of acceleration.

Pascal law states that a pressure change at any point in a confined incompressible fluid is transmitted throughout the fluid such that the same change occurs everywhere.

A well known application of Pascal's law is the hydraulic lift used to support or lift heavy objects. It is schematically illustrated in figure.

A piston with small cross section area $A_1$ exerts a force $F_1$ on the surface of a liquid such as oil. The applied pressure $P_1=\frac{F_1}{A_1}$ is transmitted through the connection pipe to a larger piston of area $A_2$. The applied pressure is the same in both cylinders as both of them are at same height, so

$$ P=\frac{F_1}{A_1}=\frac{F_2}{A_2} \text { or } F_2=\frac{A_2}{A_1} \cdot F_1 $$

Now, since $A_2>A_1$, therefore, $F_2>F_1$.