1. Angular displacement: $\theta=\frac{\operatorname{arc}}{\text { radius }}$

2. Average angular velocity: $\omega_{\mathrm{av}}=\frac{\Delta \theta}{\Delta t}$

3. Instantaneous angular velocity: $\omega=\lim \limits_{\Delta t \rightarrow 0} \frac{\Delta \theta}{\Delta t}=\frac{d \theta}{d t}$

4. Relation between linear speed and angular speed: $v=r \omega$

5. Average angular acceleration: $\alpha_{a v}=\frac{\Delta \omega}{\Delta t}$

6. Instantaneous angular acceleration: $\alpha=\lim \limits _{\Delta t \rightarrow 0} \frac{\Delta \omega}{\Delta t}=\frac{d \omega}{d t}$

7. Centripetal acceleration: $a_c=r \omega^2=\frac{v^2}{r}$

8. Centripetal force $\left(F_C\right)=m a_C=\frac{m v^2}{r}=m r \omega^2$

Note : Remember $\frac{m v^2}{r}$ is not a force itself. It is just the value of the net force acting along the inside normal (towards the centre) which is responsible for circular motion.

$\omega=\omega_0+\alpha t$

$\theta=\omega_0 t+\frac{1}{2} \alpha t^2$

$\omega^2=\omega_0^2+2 \alpha \theta$

Centripetal force $\left(F_C\right)=m a_C=\frac{m v^2}{r}=m r \omega^2$

Tangential force $\left(F_T\right)=M a_T=M \frac{d v}{d t}=M r \alpha$

where $\alpha$ is the angular acceleration.

Its magnitude is equal to that of the centripetal force i.e.,

$$ \left|F_{\text {centrifugal }}\right|=\frac{m v^2}{r}=m r \omega^2 $$

Note :

(a) Direction of centrifugal force, it is always directed radially outward.

(b) Centrifugal force is a fictitious force which has to be applied as a concept only in a rotating frame of reference to apply Newton's law of motion in that frame.

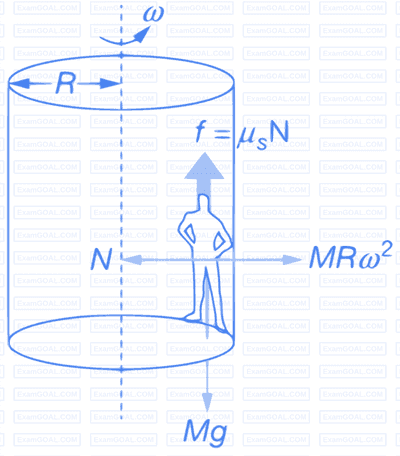
The person will remain in contact with the wall only if

Minimum speed of rotor, $\omega_{\min }=\sqrt{\frac{g}{\mu_s R}}$

If $v$ is the linear speed of rotor, then

$$ v=R \omega=\sqrt{\frac{g R}{\mu_s}} $$

$$ R \sin \theta=\frac{m v^2}{r} $$........(1)

and $R \cos \theta=m g$ .......(2)

Dividing (1) by (2), we get

$$ \tan \theta=\frac{v^2}{r g} $$

For less bending of cyclist, his speed $v$ should be smaller and radius $r$ of circular path should be greater. If $\mu$ is coefficient of friction, then for no skidding of cycle (or overturning of cyclist)

$$ \mu \geq \tan \theta=\frac{v^2}{r g} $$

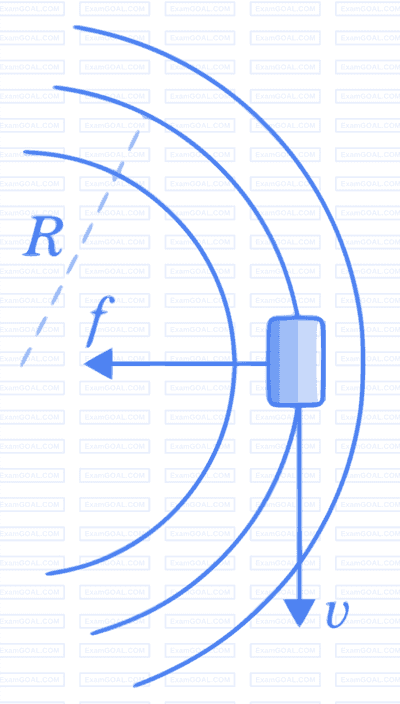
For vertical equilibrium we can write the following equation:

$$ N=m g $$ ......(1)

For circular motion in horizontal plane we can write the following equation:

$$ f=\frac{m v^2}{R} $$ ........(2)

For circular turn without slipping we can write the following equation:

$f=\frac{m v^2}{R} \leq \mu N$

$\Rightarrow \frac{m v^2}{R} \leq \mu m g$

$\Rightarrow v \leq \sqrt{\mu g R}$

So, maximum speed for no skidding is

$ v_{max} = \sqrt{\mu g R}$

$$ f_1=\frac{m v^2}{R} $$

Along the tangent :

$$ f_2=m a $$

For vertical equilibrium we can write the following equation:

$N=m g$.

From equations (1) and (2) we can write total friction acting on the car as follows:

$$ \begin{aligned} &f=\sqrt{f_1^2+f_2^2} \\\\ &f=m \sqrt{a^2+\frac{v^4}{R^2}} \end{aligned} $$

For no slipping condition while moving on circular path, we can write the following equation :

$f=m \sqrt{a^2+\frac{v^4}{R^2}} \leq \mu N$

$\Rightarrow m \sqrt{a^2+\frac{v^4}{R^2}} \leq \mu m g$

$\Rightarrow v \leq\left[\left(\mu^2 g^2-a^2\right) R^2\right]^{1 / 4}$

$N\sin \theta =\frac{m{v}^2}{r}$ .......(1)

and $N\cos \theta =Mg$ ........(2)

Dividing equation (1) by (2), we get

$\tan \theta =\frac{v^2}{rg}$

$\Rightarrow$ $v=\sqrt{rg\tan \theta }$

(a) friction $f$ will be outwards if the vehicle is at rest $v=0$. Because in that case the component of weight $m g \sin \theta$ is balanced by $f$.

(b) friction $f$ will be inwards if

$$ v>\sqrt{r g \tan \theta} $$

(c) friction $f$ will be outwards if

$$ v<\sqrt{r g \tan \theta} \text { and } $$

(d) friction $f$ will be zero if

$$ v=\sqrt{r g \tan \theta} $$

(e) for maximum safe speed (shown in figure (ii))

$$ \begin{aligned} &N \sin \theta+f \cos \theta=\frac{m v^2}{r} \\\\ &N \cos \theta-f \sin \theta=m g \end{aligned} $$

As maximum value of friction

$$ \begin{aligned} &f=\mu N \\\\ &\Rightarrow \frac{\sin \theta+\mu \cos \theta}{\cos \theta-\mu \sin \theta}=\frac{v^2}{r g} \\\\ &\Rightarrow v_{\max }=\sqrt{\frac{r g(\tan \theta+\mu)}{(1-\mu \tan \theta)}} \\\\ &\text { Similarly, } v_{\min }=\sqrt{\frac{r g(\tan \theta-\mu)}{(1+\mu \tan \theta)}} \end{aligned} $$

If $T$ be the tension in the string, then its vertical component $T \cos \theta$ balances the weight $m g$ of the bob, i. e.,

$$ T \cos \theta=M g $$

The horizontal component $T \sin \theta$ acts towards the centre of the circle and provides the centripetal force necessary for the revolution of the bob, i.e.,

$$ T \sin \theta=\frac{M v^{2}}{r} $$

where $v$ is the linear velocity of the bob along the tangent to the circle.

Dividing eqn. (ii) by (i), we get

$$ \tan \theta=\frac{v^{2}}{r g}=\frac{\omega^{2} r}{g} $$

where $\omega$ is the angular velocity of the bob.

$$ \therefore \omega=\sqrt{\frac{g \tan \theta}{r}} $$

If $T$ be the period of revolution of the bob, then

$$ T=\frac{2 \pi}{\omega}=2 \pi \sqrt{\frac{r}{g \tan \theta}} $$

From figure, $l \sin \theta=r$

$$ \therefore T=2 \pi \sqrt{\frac{l \sin \theta}{g \tan \theta}}=2 \pi \sqrt{\frac{l \cos \theta}{g}} $$

If $\theta$ be very small, then $\cos \theta=1$. So, $T=2 \pi \sqrt{\frac{l}{g}}$.

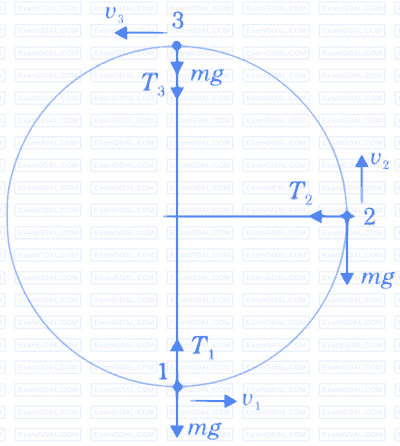
At the point $3$, both the tension $T_{3}$ and the weight $m g$ of the body act towards the centre of the circle. So $T_{3}+m g$ provides the centripetal force necessary for the rotation of the body.

$$ \therefore T_{3}+m g=\frac{m v_{3}^{2}}{r} $$

At the point $1$, the tension $T_{1}$ acts vertically upwards i.e., towards the centre of the circle and the weight $m g$ of the body acts vertically downwards i.e., in an opposite direction. So $T_{1}-m g$ provides the necessary centripetal force here.

$$ \therefore T_{1}-m g=\frac{m v_{1}{ }^{2}}{r} $$

If the tension in the string just vanishes at $3$ i.e., if $T_{3}=0$, then

$$ m g=\frac{m v_{3}^{2}}{r} \text { or, } v_{3}=\sqrt{g r} $$

If the velocity of the body at the highest point $3$ be less than $\sqrt{g r}$, the string will slack and the body will drop down instead of rotating in the circular path. So this minimum velocity of the body at the highest point is called the critical velocity.

Minimum velocity at the lowest point for maintaining the critical velocity :

Now, as the body goes from $3$ to $1$, its height increases by $2 r$. So its potential energy increases by $m g \times 2 r$. From the principle of conservation of mechanical energy, we have

The K.E. of the body at $3$ - Its K.E. at $1=$ Increase in P.E.

or, $1 / 2 m v_{3}^{2}-1 / 2 m v_{1}^{2}=2 m g r$

or, $v_{3}^{2}=v_{1}^{2}+4 g r$

When $v_{3}=\sqrt{g r}, v_{1}$ is minimurn

$\therefore\left(v_{1}\right)_{\min }=\sqrt{g r+4 g r}=\sqrt{5 g r}$

Minimum tension :

When the body moves with the critical velocity at the highest point, the tension in the string becomes zero; then $m g=\frac{m v_{3}^{2}}{r}$ When this condition is satisfied, the tension in the string at the lowest point $1$ becomes minimum.

So, $$ \left(T_{1}\right)_{\min }=\frac{m\left(v_{1}\right)^{2} \min }{r}+\frac{m\left(v_{3}\right)^{2} \min }{r}=\frac{m}{r}(5 g r+g r)=6 \mathrm{mg} $$

Relative angular velocity of a particle 'A' w.r.t. other moving particle B is the angular velocity of the position vector of A w.r.t. B.

That means it is the rate at which position vector of 'A' w.r.t. B rotates at that instant.



$\omega_{A B}=\frac{\left(v_{A B}\right)_{\perp}}{r_{A B}}=\frac{\begin{array}{l}\text { Relative velocity of } A \text { w.r.t. } \\ B \text { perpendicular to line } A B\end{array}}{\text { seperation between } A \text { and } B}$

here $\left(v_{A B}\right)_{\perp}=v_A \sin \theta_1+v_B \sin \theta_2 $

$\therefore \omega_{A B}=\frac{v_A \sin \theta_1+v_B \sin \theta_2}{r}$