Stress
Strain
Longitudinal strain
Volume strain
Shear strain,
Hooke's Law
Stress strain
stress = k strain
where k is the proportionality constant and is known as modulus of elasticity.
Young's Modulus of Elasticity
If is the length of wire, is radius and is the increase in length of the wire by suspending a weight Mg at its one end then Young's modulus of elasticity of the material of wire
Increment in Length Due to Own Weight
Bulk Modulus of Elasticity
Bulk modulus of an ideal gas is process dependent.
- For isothermal process, $\mathrm{PV}=$ constant
$$ \Rightarrow \mathrm{PdV}+\mathrm{VdP}=0 \Rightarrow P=\frac{-\mathrm{dP}}{\mathrm{dV} / \mathrm{V}} $$
So bulk modulus $=P$
- For adiabatic process $PV^\gamma=$ constant
$$ \begin{aligned} &\Rightarrow \gamma \mathrm{PV}^{\gamma-1} \mathrm{dV}+\mathrm{V}^\gamma \mathrm{dP}=0 \\\\ &\Rightarrow \gamma \mathrm{PdV}+\mathrm{VdP}=0 \Rightarrow \gamma \mathrm{P}=\frac{-\mathrm{dP}}{\mathrm{dV} / \mathrm{V}} \end{aligned} $$
So bulk modulus $=\gamma \mathrm{P}$
- For any polytropic process $\mathrm{PV}^{\mathrm{n}}=$ constant
$$ \begin{aligned} &\Rightarrow \mathrm{nPV}^{\mathrm{n}-1} \mathrm{dV}+\mathrm{V}^{\mathrm{n}} \mathrm{dP}=0 \Rightarrow \mathrm{PdV}+\mathrm{VdP}=0 \\\\ &\Rightarrow \mathrm{nP}=\frac{-\mathrm{dP}}{\mathrm{dV} / \mathrm{V}} \end{aligned} $$
So bulk modulus $=n P$
Compressibility
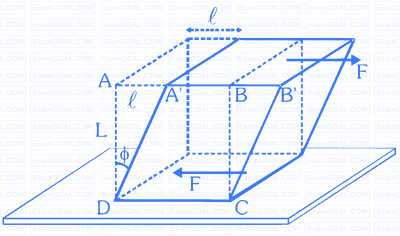
Modulus of Rigidity
Poisson's Ratio
Let a bar be of length l and diameter d. When a tensile force is applied along the length of the bar, the length increases l + l and the diameter decreases d d.
lateral strain =
Longitudinal strain =
Poisson's ratio of bar components
Relationship between density change and Bulk Modulus
If pressure increases P + dP, then density becomes + d and volume becomes V + dV.
Bulk Modulus,
Relationship Between Longitudinal Strain and Volume Strain
Let a bar of circular cross-section be of length l and radius r.
Bar volume
by differentiating we get,
or,
[ ]
If Poisson's ratio of bar components is , then
or,
or,
Volume Strain = Longitudinal Strain (1 2 Poisson's ratio)
Note : Poisson's ratio under linear deformation of an object where volume deformation is negligible i.e. volume remains almost unchanged is = 0.5
If volume deformation is negligible compared to linear deformation, then
or, .
Correlation Between Elastic Constants
Among them the following two relations can be proved,
...... (1)
...... (2)
Doing (1) + (2),
or,
or,
Doing (1) (2) ,
or,
or,
or,
TORSION CONSTANT OF A WIRE
(a) Toque required for twisting by an angle .
(b) Work done in twisting by an angle .